In a piece I authored in June 2018 (Sacco, 2018), I introduced a technique designed to forecast synchronistic events, be it within counseling sessions or for personal introspection. By employing this approach, individuals can attain a more profound comprehension of the synchronicities that have colored both their past and present.

In this blog post, I'll further unpack the nuances of this technique, which I've called the "Harmonic Model."

Carl Jung (1952) characterized synchronicity as the consequential overlap between external and internal occurrences. He refined his perspective on synchronicity in collaboration with the renowned quantum physicist, Wolfgang Pauli.

Synchronicities stand apart as acausal events, meaning they don't align with traditional cause-and-effect paradigms. Jung coined the term "synchronicity" as a canopy concept, encapsulating various paranormal instances within its ambit. These events often defy regular causality, and are occasionally labeled as superstitious, magical, or supernatural.

"Synchronization" references the spontaneous manifestation of order in intricate systems, marked by distinct geometric patterns and an almost periodic structure (Pikovsky, Rosenblum, & Kurths, 2001). Notably, contemporary research underscores the pivotal roles of Fibonacci numbers and the golden ratio in synchronization.

The Fibonacci sequence (1, 1, 2, 3, 5, 8, 13, 21, and so on) evolves recursively, where each number is the summation of its two predecessors. An intriguing mathematical relationship emerges between the Fibonacci sequence and the golden ratio (approximately 1.618034). As one progresses through the Fibonacci numbers, the ratio of successive numbers tends to converge on the golden ratio.

Delving deeper into the enigma of synchronicity, the path forward lies in crafting theories that demystify the origins of such experiences. Since Jung's introduction of the synchronicity theory (Jung, 1952), scholars have grappled with conceptualizing a comprehensive model for this phenomenon.

Drawing from Sacco's works (2016, 2018), it's posited that Fibonacci numbers hold the potential to forecast synchronistic events (Sacco, 2016). In the ensuing section, I will detail the methodology crafted to realize this intriguing proposition.

The Harmonic Model offers a robust framework for predicting the phenomena of synchronicity. By grasping the origin and nature of synchronistic events, researchers and clinicians can more effectively harness transformative processes.

Central to this model is the role of Fibonacci numbers. These numbers, which permeate from the quantum realm to DNA structures, biological cell divisions, and even self-organizing systems, serve as powerful predictors of both physical and psychological shifts. Notably, they shed light on increases in synchronistic experiences.

Employing a computer-assisted approach, the Harmonic Model forecasts synchronicity throughout human life. The methodology involves:

1. Inputting a birthdate;
2. Computing primary intervals by augmenting the birthdate with the initial 21 Fibonacci numbers, where each Fibonacci number equates to a 24-hour timeframe;
3. Deriving secondary intervals, which employ dates from the previous step to establish standing wave harmonics. These harmonics manifest when the primary intervals recur consistently up to age 78.51 or another predetermined endpoint;
4. Representing these intervals graphically.

For counselors, the Harmonic Model becomes a tool of insight and guidance:
​
1. They plot the computed time intervals;
2. Analyze the ensuing graphical representation; and 
3. Provide counsel tailored to the insights derived from the model.

Harmonic Model-based counseling offers a holistic methodology, integrating diagnostic components with clear guiding principles for interventions.

Illustrative figures provided alongside further elucidate these advantages and the model's broader implications.

Figure 1 presents the primary interval calculations based on a birthdate of January 1, 2000 (depicted as 102). Here's a breakdown:

1. The Fibonacci sequence displays its first 21 numbers, labeled as (100).
2. In the stage marked by 101, these initial 21 Fibonacci numbers are translated to correspond with a 24-hour clock format.
3. Step 102 involves adding these Fibonacci numbers to the individual's birthdate.
4. The subsequent age accumulation, expressed in years, is illustrated in step 103.

Figure 2 showcases the secondary interval computations of the Harmonic Model, which are built upon the primary interval calculations (represented as 103). Here's an elucidated breakdown:

1. The secondary date calculations, denoted as (200-208), stem from the final nine primary interval computations (104-112).
2. These secondary intervals are obtained by adding the primary intervals, beginning from the birthdate.
3. To illustrate, the computation for the secondary interval on 2003-05-03 [210] is derived from 2001-09-1 [209] by adding 1.67 years (sourced from 104). 
4. These calculations exemplify the nodal points characteristic of standing wave harmonics.
5. The antinodes, marked as [211], are computed using the mean values of the neighboring nodes.

The Fibonacci Lifechart presents the secondary interval computations in two formats: a cycle plot, as depicted in Figure 2, and a sequential listing. By juxtaposing both the cycle plot and the chronological list of dates against the seven life domains (namely residence, cohabitation, intimate relationships, family, occupation, health, and spiritual experience), one can assess instances of synchronicity experiences.​
​Click here to get a copy of the Fibonacci Lifechart. 

Coldea, R., Tennant, D. A., Wheeler, E. M., Wawrzynska, E., Prabhakaran, D., Telling, M.,... Kiefer, K. (2010). Quantum criticality in an Ising chain: Experimental evidence for emergent E8 symmetry. Science, 327(5962), 177-180. http://sci-hub.tw/10.1126/science.1180085

Jung, C. G. (1952). Synchronicity: An acausal connecting principle. CW 8.

Pikovsky, A., Rosenblum, M., & Kurths, J. (2001). Synchronization: A universal concept in nonlinear sciences. Cambridge, UK: Cambridge University Press.

Pletzer, B., Kerschbaum, H., & Klimesch, W. (2010). When frequencies never synchronize: The golden mean and the resting EEG. Brain Research, 1335, 91-102. http://sci-hub.tw/10.1016/j.brainres.2010.03.074

Sacco, R. G. (2016). The Fibonacci Life-Chart Method (FLCM) as a foundation for Carl Jung’s theory of synchronicity. Journal of Analytical Psychology, 61(2), 203-222. http://sci-hub.tw/10.1111/1468-5922.12204

Sacco, R. G. (2018). Fibonacci harmonics: A new mathematical model of synchronicity. Applied Mathematics, 9, 702-18. http://sci-hub.tw/10.4236/am.2018.96048

Sacco, R.G. (2019). Modeling celestial mechanics using the Fibonacci numbers. International Journal of Astronomy, 8, 8-12. http://sci-hub.tw/10.5923/j.astronomy.20190801.02

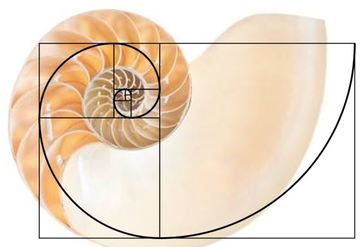
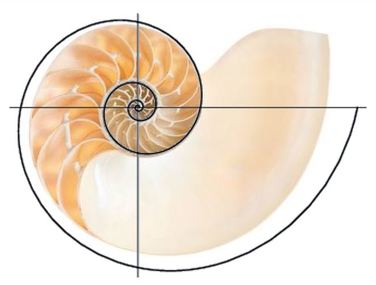
The Fibonacci sequence is a marvel that has captivated mathematicians throughout history. But its allure extends beyond math, influencing fields like art, biology, architecture, music, botany, and finance.

Is this truly the world's most mesmerizing number sequence, or are we stretching our imagination to find patterns where none lie?

Join us as we delve deep into this mathematical wonder that has fascinated minds across disciplines and eras.

1. Does Architectural design reflect the golden ratio?

The golden ratio is often touted as appearing in many architectural marvels, both ancient and contemporary. Examples span from the Great Pyramid of Giza and the Parthenon to modern landmarks like the Eiffel Tower, Toronto’s CN Tower, and the United Nations Secretariat building.

It's debated whether all these structures consciously employ the golden ratio in their designs. The Parthenon, with its intricate layout, leaves us guessing about its architects' intentions regarding the golden ratio.

However, there seems to be a deliberate use of the golden ratio in the design of Toronto’s CN Tower. The proportion between its total height (553.33 meters) and the height to its observation deck (342 meters) is strikingly close to 1.618, the golden ratio.​​

2. Is the spiral of the Nautilus shell based on the golden ratio?

Indeed, there's more nuance than popular narratives suggest. The classic "golden spiral" is crafted using consecutive golden rectangles, resulting in a spiral that expands by the golden ratio for every quarter turn. However, the Nautilus shell's spiral isn't an exact match to this golden spiral.

Here's the crux of the matter: The golden ratio can shape spirals in multiple ways. 

For instance, a spiral that widens according to the golden ratio after every 180-degree twist more closely mirrors the spirals found in numerous Nautilus shells.

I trust you grasp the distinction.

Here's another intriguing insight: The nautilus shell expands according to the number 108 (refer to "The Number 108"). This number resonates with the pentagram, which fundamentally operates on the principles of the golden ratio.

Thus, it's evident that the Nautilus spiral can indeed reflect proportions approaching Phi.

3. Did renaissance artists use the golden ratio in their paintings?

Absolutely. Leonardo Da Vinci's "Last Supper" prominently features golden ratios.

Inspired by him, Salvador Dalí, in 1955, crafted "The Sacrament of the Last Supper" adhering to golden ratio dimensions.

Similarly, in Michelangelo’s "Creation of Adam," the iconic touch between God’s and Adam’s fingers occurs exactly at the golden ratio point of the width within their frame.

These instances highlight just a fraction of the Renaissance artists' fascination with the golden ratio in their masterpieces.

4. Are the spirals seen in nature based on the golden ratio?

Spirals in nature are often linked to the golden ratio, and while it's not universally true, there are noteworthy instances.

Sunflower seeds, for instance, form interconnecting spirals based on Fibonacci numbers. This Fibonacci-driven pattern maximizes the number of seeds on a seed head.

Beyond sunflowers, the golden ratio influences the growth of leaves, branches, and petals, optimizing sunlight absorption as new leaves emerge.

Nature frequently showcases logarithmic spirals: from spiral galaxies and ram horns to hurricanes and whirlpools. The golden spiral is a subset of these logarithmic spirals, and while it may be present in the examples listed, not all natural logarithmic spirals are golden spirals.​

5. Is there a new algorithm based on the golden ratio that can predict spiritual experience?

It seems that there might be a connection between mathematics and spiritual experience. Sacco's 2017 study tentatively suggested that the dynamic effects observed at age 18 could potentially predict an elevation in spiritual experiences. This observation aligns with the predictions of the FLCM. This offers a tentative link between the relationship of the golden ratio and spiritual experience.
​
However, the dynamical effects of age 11 and 30 did not support the hypothesis of increased spiritual experience as predicted. This result required an alternative explanation. 

It should be evident that children at age 11 may not be able to communicate the complexities of some spiritual experiences. And it’s entirely reasonable that spiritual experiences could be linked with the instability that is less likely at age 30 when personality becomes stabilized.

In the real world, there are many compelling examples of the golden ratio in natural phenomena. But not all phenomena in nature are based on the golden ratio. 

You can find plenty of examples where the golden ratio is not found in nature as might be expected. But you can’t deny the power of data and evidence to prove the inherent power of the golden ratio in reality.

The critical question you need to ask yourself is: Why does the golden ratio show up so commonly in nature? 

The Golden Ratio: A Principle of Energy Flow

The golden ratio, seen in structures as vast as galaxies or as intricate as DNA, has long been the symbol of ideal harmony. Duke University's Adrian Bejan ties this unique ratio to a universal law of nature's design. Through his work on the constructal law, Bejan reveals how nature shapes itself to ease flow. The essence of the golden ratio, he suggests, lies in achieving maximal efficiency with minimal energy (Bejan, 2009).

This ratio allows structures to scale infinitely without changing their core shape, resulting in its recurrence throughout nature. By choosing the path of least resistance, the golden ratio epitomizes energy-efficient flow. This is evident in falcons, which harness the golden spiral for an energy-saving approach to their prey (Tucker, 2000), and in plants, where Fibonacci spirals display energy optimization in phyllotaxis (Li et al., 2007).

​The Purpose of Life: Flow

The Constructal Law intertwines modern science with ancient spiritual writings, hinting at a unified purpose for all existence: to facilitate energy flow. Energy naturally seeks equilibrium, flowing from concentrations, like the sun, to the expansive universe.

Our individual purpose is deciphering our unique energy flow. Despite being made of the same elements, each person's energy interplays differently. Our brains might share a similar number of nerves, but the connections, molded by experiences, are distinct, crafting our personalities.

When our energies align, we enter a "flow" state, a concept explored by Csikszentmihalyi (1990) and Maslow (1964). In this state:

Causes of Flow:

- Clear goals
- Immediate performance feedback
- Confidence in handling challenges

Characteristics of Flow:

- Absolute focus
- Control and openness
- Enhanced learning and positivity

Consequences of Flow:

- Transcendence of self-awareness
- Altered time perception
- Intrinsic reward from the activity

Discovering your energy's optimal flow—whether through passions or talents—uncovers your unique life purpose. Like fingerprints, our energy patterns are singular. Aligning with this flow often leads to peak performance, happiness, and fulfillment, underscoring our individual roles in the universe.

References
​
Bejan, A. (2009). The golden ratio predicted: Vision, cognition and locomotion as a single design in nature. International Journal of Design & Nature and Ecodynamics, 4(2), 97-104.

Csikszentmihalyi, M. (1990). Flow: The Psychology of Optimal Experience. Harper and Row, New York, NY.

Li, C., Ji, A., & Cao, Z. (2007). Stressed Fibonacci spiral patterns of definite chirality. Applied Physics Letters, 90(16), 164102.

Maslow, A. H. (1964). Religions, values, and peak-experiences. Columbus, OH: Ohio State University Press. (Original work published 1940)

Tucker, V. A. (2000). The deep fovea, sideways vision and spiral flight paths in raptors. Journal of Experimental Biology, 203(24), 3745-3754.

The Sacred Significance of "108"

The number "108" holds profound significance across various spiritual traditions. In Hinduism and Buddhism, it represents a path of balance with 108 virtues to nurture and 108 defilements to shun. This sense of equilibrium extends to their malas (or rosaries), which feature 108 beads. In Islamic traditions, "108" is sometimes invoked in place of God's name. Even in tai chi, a practice rooted in fluid motion and balance, there are 108 movements. The recurring presence of this number across diverse spiritual practices suggests its importance goes beyond mere coincidence.

108 and Growth

​The number 108 has intriguing ties to the cosmos: the average distance from the Sun and Moon to Earth is 108 times their respective diameters. Beyond this celestial connection, the natural world showcases 108 in the growth of a nautilus shell. As the nautilus matures, each new chamber of its shell expands to be 1.08 times larger than the previous one, crafting a mesmerizing logarithmic spiral on the shell's exterior.

In the BBC Two documentary "The Code," Professor Marcus du Sautoy from the University of Oxford unveils the elegant spiral shell of the nautilus, which grows at a constant rate of 1.08. He delves into how spirals and the wonders of math manifest across nature.

108 and The Number 5
​
​​The number 108 is related to the number 5. The clue is related to the angle of the Pentagon, being 108˚.​

​108 and The Fibonacci Sequence
​
The number 108 has a profound significance when looked through the lens of decimal parity, an ancient numerical system used by cultures like Egypt and India to discern the essence of numbers.

Decimal parity simplifies numbers to single digits. Take 361: 3 + 6 + 1 = 10, and further, 1 + 0 = 1. Thus, 361's decimal parity is 1.

Consider the Fibonacci Sequence's first 24 numbers: 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, 233, 377, 610, 987, 1597, 2584, 4181, 6765, 10946, 17711, 28657.

Applying decimal parity to this sequence yields a recurring pattern of 24 digits: 0, 1, 1, 2, 3, 5, 8, 4, 3, 7, 1, 8, 9, 8, 8, 7, 6, 4, 1, 5, 6, 2, 8, 1. The sum? A familiar 108.

Intriguingly, this pattern mirrors the 1.08 growth constant the nautilus adopts for its spiral shell. The connection between nature's designs and ancient numerical systems is truly fascinating.